
The diagram below is something called the 'Circle of Fifths'. It's a bit daunting at first sight, but it's well worth spending some time looking at.
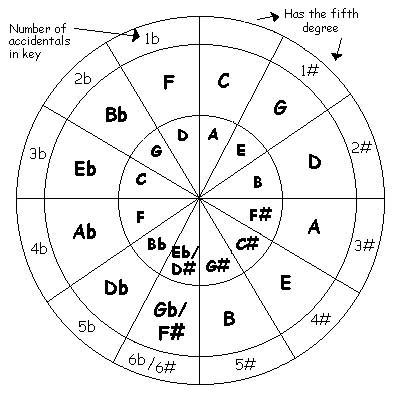
Let's start with the outer band of notes, starting with the top. This represents the key of C major, which has no accented notes in it (C, D, E, F, G, A, B - hence the empty 'cell' on the very outer rim of the circle next to the C. We can move clockwise around the circle in steps of seven semitones (or a perfect fifth, hence the name). Why a fifth? Well, check this out...
The fifth degree of C major is G. Taking the same set of intervals that we used t build a major scale on the note of C, and arranging them from a G note gives us a G major scale. This scale has the notes G, A, B, C, D, E and F# - only one note name different from C major. This single accented note is represented in the outer cell of the circle with the 1#.
Now move up by a further perfect fifth. A perfect fifth up from G is D, which has the major scale D, E, F#, G, A, B and C# - all the notes from the G major scale, albeit in a different order, but with a C# instead of a C. Two accented notes, hence the '2#' in the outer cell of the circle.
And that's the general rule - to get the notes of a major scale, take the notes of the major scale a perfect fifth below, re-arrange the note names, and accent the seventh degree of the new scale with a # sign. This works fine right the way around the circle until we get to F# major. You can actually extend it further to get to C# major (7 accented notes) but that's not shown here.
Making sense so far? Then let's go back to C major and start working anti-clockwise. Same procedure here, but we'll step down an interval of a perfect fourth (a descending perfect fourth is the inverse of an ascending perfect fifth. I know that there isn't a tutorial on the website yet about inversions of intervals, but you should be able to work this one out - contact me for more info if you need it). Some people will refer to the Circle of Fifths as the 'Cycle of Fourths' but they're talking about the same thing.
A perfect fourth down from C is F. The F major scale has the notes F, G, A, Bb, C, D and E. A perfect fourth down from F is Bb (Bb major = Bb, C, D, Eb, F, G, A). See the pattern emerging?
The rule here is this - to get the notes of a major scale, take the notes of the major scale a perfect fourth above, re-arrange the note names and accent the fourth degree of the new scale with a b sign. This works fine through to Gb major (6 flats, shown on the circle).
Recently, I received an email from a visitor to this site, asking "what about C#?" For some reason, traditionally neither C# major (A# natural minor) or Cb major (Ab natural minor) appear on the circle of fifths diagram. I presume that this is beacuse they could detract from the symmetry of the diagram. These keys do exist though, with 7 shaprs and 7 flats respectively.
The same pattern works for the natural minor keys, which are shown here in the inner segments of the circle. Each part of the circle shows a major key plus its relative natural minor key. If you're new to the natural minor scale, then there is another tutorial which you might find useful.
Notice how many notes there are in common between adjacent keys on the circle - 6 notes out of seven are the same. If you want to write a key change into a piece of music, then moving one step around the Circle of Fifths is a good direction to go.
I often advise incorporating the Circle of Fifths into your daily practise routine. For example, play through the major scale starting with C major, and do a full trip around the Circle - much more fun that just stepping up a semitone at a time and playing C major, Db major, D major, Eb major, etc. You can repeat the pattern with every scale that you know - but more about daily practise routines in a future tutorial.
How useful did you find this tutorial?
| Product/Info... | PhatPhish Application | | | Product Help | | | GUPPY - PhatPhish for the web | | | About The Author |
|---|
| Get Stuff... | Download PhatPhish | | | Mechandise | | | Blank Stave And Tab Sheets | | | Tutorials |
|---|
| Do Stuff... | Register | | | Feedback | | | Links | | | Donate | | | Ask A Question |
|---|
| Social Media... | YouTube | | | | |
|---|
| Promote... | Spread The Word | | | Posters/Flyers |
|---|
| Play... | PhatPhish Picks - Boutique Plectrums |
|---|
| ©2002-2022, Dave Dixon / CyberFlotsam http://www.cyberflotsam.com |